There is a beautiful mathematical property that relates multiplication and addition. It is called the associative property of multiplication, and it states that for any two multiplicands, the product is also a multiplicand. In other words, if you multiplicative two numbers together and then add the result to another number, the new number will be associated with the first two numbers similarly. For example, 3x+4 is associated with 3+4, 5x+6 is associated with 5+6, and so on.
What is an example for associative property?
One example for associative property is the multiplication of numbers. For example, if you multiply 2 by 3, the result is 7. However, if you multiply 3 by 2, the result is 6. This is because the order of operations (or operator precedence) determines which operation to carry out first.
What is associative and commutative property of multiplication?
The associative and commutative property of multiplication states that the order of operations (also called precedence, or algebraic order) for multiplying two numbers is: left-to-right, then right-to-left. This means that if you have a number written like this: 3×4, then the order of operations is: 3, 4, x.
What is the associative property of multiplication worksheets?
The associative property of multiplication states that if multiplication worksheets are associative, then the product of two multiplicands is also associative. In other words, the order of operations (left-to-right) does not affect the associativity of multiplication.
This associative property is illustrated in the following worksheet. On the left side, multiply 3 by 2 and on the right side, multiply 5 by 2. The product, 12, is not associative because it depends on the order of operations.
What are the properties of associative?
The associative property is a mathematical property that states that a multiplication operation on two numbers is commutative. In other words, the order of the multiplication doesn’t affect the result. For example, 3×2 = 6 and 2×3 = 6.
What are the 4 types of multiplication properties?
There are four types of multiplication properties: commutative, associative, distributive, and associative distributive.
Commutative property: Multiplication is commutative – the order of the operations does not affect the result. For example, 3 x 4 = 12.
Associative property: Multiplication is associative – the order of the operations does not affect the result, except for the order of parentheses. (3 x (4 + 5)) = 12. This is because (3 x 4) + (3 x 5) = 12.
Distributive property: Multiplication is distributive – the result of a multiplication is distributed among the operands according to the rule (a + b)n = an+bn. For example, 3 + 4 = 7. 5n+6 = 35.
Associative distributive property: Multiplication is associative distributive – the result of a multiplication is distributed according to both the rule (a + b)n=an+bn and the rule (c + d)n=cd+dn. For example, (3 + 4)x5=15 and 9×6= 45.
Why would you use the associative property of multiplication?
The associative property of multiplication is a mathematical property that states that the product of two numbers is the same as the sum of their multiplications. This is often used when working with complex algebraic expressions. For example, the product 3x + 4 is equal to 3x + 4x because both 3 and 4 are multiplied before adding them together.
How do you write associative property?
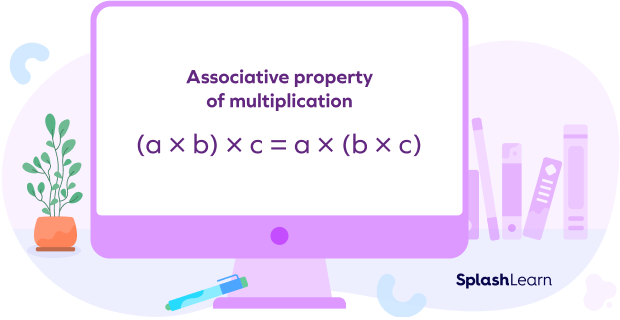
The associative property of multiplication states that if a, b, and c are any three numbers, then (a*b) * c is equal to a*(b*c), regardless of the order in which they are multiplied. This property can be illustrated by considering the following equation:
3x + 2y = 10
Because 3x and 2y are associated, multiplying both sides by 3 results in a net result of 11. In the same way, multiplying y by 2 on the left side results in 6, and multiplying x by 1 on the right side results in 3. The associative property of multiplication is always true.
What is an associative property in math?
An associative property in math is a rule that states that if two mathematical operations are associative, then their products are also associative. For example, the multiplication table (from left to right) can be thought of as an associative property:
3 × 2 = 6
5 × 4 = 15
7 × 6 = 43
This means that when multiplying two numbers together, the order of operations doesn’t matter – the product will always be the same. The distributive property is also an associative property:
(a + b) c = ac + bc
This means that if you add two numbers together, the order of operations doesn’t matter – the result will always be the same.
What is associative property with Example Class 7?
Associative property of multiplication is a mathematical property which states that the associative law holds true for all multiplication. It is also known as distributive law of multiplication. This law states that for every product A ⊕ B there exists another product C such that A(C) = B(C).
In other words, the following statement is always true:
AB = BA
There are many examples where this law can be used to simplify complex algebraic expressions. For example, let’s consider the example class 7. Here, we have two elements – 1 and 7. The class 7 can be seen as the product of these two elements. So, 1(7) = 7(1), and 2(7) = 14(1). In other words, the distributive law of multiplication helps us to simplify our calculations quickly.